Laboratory work “Determination of spring stiffness” educational and methodological material in physics (grade 7)

• home
• Directory
• Physics

When exposed to external forces, bodies are capable of acquiring acceleration or deformation.
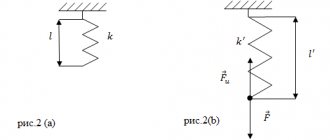
Deformation is a change in the size and (or) shape of a body. If, after removing the external load, the body restores its size and shape completely, then such deformation is called elastic. Let the spring in Fig. 1 be acted upon by a tensile force directed vertically downward.

When exposed to a deforming force ($\overline{F}$), the length of the spring increases. An elastic force (${\overline{F}}_u$) arises in the spring, which balances the deforming force. If the deformation is small and elastic, then the elongation of the spring ($\Delta l$) is proportional to the deforming force:

\[\overline{F}=k\Delta l\left(1\right),\]

where the proportionality coefficient is the spring stiffness $k$. The coefficient $k$ is also called the elasticity coefficient, the stiffness coefficient. Stiffness (as a property) characterizes the elastic properties of a body subjected to deformation - this is the body’s ability to resist external force and maintain its geometric parameters. The stiffness coefficient is the main characteristic of stiffness.

The spring stiffness coefficient depends on the material from which the spring is made and its geometric characteristics. Thus, the stiffness coefficient of a twisted cylindrical spring, which is wound from round wire, subjected to elastic deformation along its axis, is calculated using the formula:

\[k=\frac{Gd^4}{8d^3_pn}\left(2\right),\]

where $G$ is the shear modulus (a value depending on the material); $d$ is the wire diameter; $d_p$ is the diameter of the spring coil; $n$ — number of spring turns.

Types of deformation

Deformation is a change in the shape or size of the body.

There are several types of deformation:

• shift;
• torsion;
• bend;
• compression/extension;

Shear deformation occurs when some parts of the body move relative to other parts of the body. If we apply a horizontal force to the top of a cardboard box filled with various objects, we will cause the top of the box to shift relative to its bottom.

Compression or stretching is easy to imagine using the example of a rectangular piece of thin rubber. This deformation is used, for example, in elastic bands for clothing.

Examples of bending and torsion are shown in Figure 1. A plastic ruler deformed by bending is shown in Figure 1. 1a, and in Figure 1b - the same ruler deformed by torsion.

Rice. 1. plastic ruler, deformed by bending – a) and torsion – b)

In a deformable body, forces arise that are of an electromagnetic nature and prevent deformation.

Sources and notes

1. ↑
   Elastic deformation (Russian). Archived from the original on June 30, 2012.
2. ↑ Dieter Meschede, Christian Gerthsen.
   Physik.
   - Springer, 2004. - P. 181
   ..
3. ↑ Bruno Assmann.
   Technische Mechanik: Kinematik und Kinetik.
   - Oldenbourg, 2004. - P. 11
   ..
4. ↑
   Dynamics, Elastic force (Russian). Archived from the original on June 30, 2012.
5. ↑
   Mechanical properties of bodies (Russian). Archived from the original on June 30, 2012.

Wikimedia Foundation. 2010.

See what “Elasticity coefficient” is in other dictionaries:

elasticity coefficient - tampros koeficientas statusas T sritis Standartizacija ir metrologija apibrėžtis Dydis, atvirkščiai proporcingas tampros moduliui. atitikmenys: engl. elasticity coefficient vok. Elastizitätskoeffizient, m rus. elasticity coefficient, m pranc.… … Penkiakalbis aiškinamasis metrologijos terminų žodynas

elasticity coefficient - tamprumo koeficientas statusas T sritis fizika atitikmenys: engl. elasticity coefficient vok. Elastizitätskoeffizient, m rus. elasticity coefficient, m pranc. coefficient d'élasticité, m … Fizikos terminų žodynas

FORMATION ELASTICITY COEFFICIENT - β* σ, which reads the elastic expansion of the liquid contained in the reservoir and the decrease in pore volume due to the elasticity of the reservoir and characterizes the elastic reserve of the reservoir system. K.u. p. is determined by the formula: βp = mβzh + βp, where m... ... Geological Encyclopedia

Spring stretch

Let's take a closer look at tensile deformation using the example of a spring.

Let's attach a spring to some surface (Fig. 2). The figure on the left shows the initial length \(L_{0}\) of the spring.

Rice. 2. By comparing the length of the free spring with the length of the loaded one, you can find its elongation

Now let's hang a weight on the spring. The spring will have the length \(L\) shown in the figure to the right.

Let's compare the length of the loaded spring with the length of the freely hanging spring.

\[ \large L_{0} + \Delta L = L \]

Let's find the difference (difference) between the lengths of a freely hanging spring and a spring with a load. To do this, subtract the value \(L_{0}\) from both sides of this equation.

\[ \large \boxed{ \Delta L = L — L_{0} }\]

\( L_{0} \left(\text{m} \right) \) – initial length of the spring;

\( L \left(\text{m} \right) \) – final length of the stretched spring;

\( \Delta L \left(\text{m} \right) \) – a piece of length by which the spring was stretched;

The quantity \(\Delta L\) is called the elongation of the spring.

Sometimes relative elongation is calculated. This elongation is often expressed as a decimal fraction. Or a fraction whose denominator is the number 100 - such a fraction is called a percentage.

Note: A ratio is a fraction. Relative means fractional.

\[ \large \boxed{ \frac{\Delta L }{ L_{0}} = \frac{ L — L_{0}}{L_{0} } = \varepsilon } \]

\( \varepsilon \) is the ratio (proportion) of the stretch of the spring to its initial length. It is measured as a percentage and is called relative elongation.

Calculation of elastic force

If we stretch a spring by hand, we can notice that the more we stretch the spring, the more it resists.

This means that the elongation of the spring is associated with a force that resists this elongation.

Of course, if the spring is elastic enough to resist. For example, a multi-colored toy spring (Fig. 3), made of plastic, will practically not resist stretching, which doubles its length.

A multi-colored plastic toy spring has little resistance to stretching.

Hooke's law

The English physicist Robert Hooke, who lived in the second half of the 17th century, established that the resistance force of a spring and its elongation are directly proportional. He called the force with which the spring resists deformation \( F_{\text{control}} \) the force of elasticity.

\[ \large \boxed{ F_{\text{control}} = k \cdot \Delta L }\]

This formula was called Hooke's law of elasticity.

\( F_{\text{control}} \left( H \right) \) – elastic force;

\( \Delta L \left(\text{m} \right) \) – spring elongation;

\( \displaystyle k \left(\frac{H}{\text{m}} \right) \) – stiffness (elasticity) coefficient.

What deformations are called small

Hooke's law is used for small elongations (strains).

If the deforming force is removed and the body returns to its original shape (size), then the deformations are called small.

If the body does not return to its original shape, the deformation cannot be called small.

How to calculate the stiffness coefficient

A weight attached to the end of the spring stretches it (Fig. 4). Let's measure the elongation of the spring and create a force equation for the projection of forces on the vertical axis. The weight of the load is directed against the axis, and the elastic force opposing it is directed along the axis.

Rice. 4. The weight of a load suspended on a spring is balanced by the elastic force

Since the forces cancel each other, there is a zero on the right side of the equation.

\[ \large F_{\text{control}} - m \cdot g = 0 \]

Let us substitute the expression for the elastic force into this equation

\[ \large k \cdot \Delta L — m \cdot g = 0 \]

Let's add the weight of the load to both parts and divide by the measured change in length \(\Delta L\) of the spring. We obtain an expression for the stiffness coefficient:

\[ \large \boxed{ k = \frac{ m \cdot g }{\Delta L} }\]

\(g\) is the acceleration of gravity, it is related to the force of gravity.

How did the first version of the formula appear?

The formula for calculating the spring stiffness, which is called Hooke's law, was established experimentally. During experiments with loads of different masses suspended on an elastic element, the magnitude of its elongation was measured. So it turned out that the same test part under different loads undergoes different deformations. Moreover, hanging a certain number of weights of equal mass showed that each added/removed weight increases/decreases the length of the elastic element by the same amount.

As a result of these experiments, the following formula appeared: kx=mg, where k is a certain constant coefficient for a given spring, x is the change in the length of the spring, m is its mass, and g is the acceleration of gravity (approximate value is 9.8 m/s²) .

This is how the property of rigidity was discovered, which, like the formula for determining the elasticity coefficient, finds the widest application in any industry.

We connect two identical springs

In physics problem books and textbooks for preparing for the Unified State Exam, there are problems in which identical springs are connected in series or in parallel.

Parallel connection of springs

Figure 5a shows a freely hanging spring. Let's load it (Fig. 5b), it will stretch by the amount \(\Delta L\). Let's connect two such springs in parallel and hang a load in the middle of the crossbar (Fig. 5c). The figure shows that a structure of two parallel springs under the action of a load will stretch less than a single such spring.

Rice. 5. Two springs connected in parallel are deformed less than one such spring

Let us compare the stretch of two identical springs connected in parallel with the stretch of one spring. We hang one load weighing \(mg\) from the springs.

One spring:

\[ \large k_{1} \cdot \Delta L = m \cdot g \]

Two parallel springs:

\[ \large k_{\text{parallel}} \cdot \Delta L \cdot \frac{1}{2}= m \cdot g \]

Since the right sides of the equations are the same, the left sides will also be equal:

\[ \large k_{\text{parallel}} \cdot \Delta L \cdot \frac{1}{2}= k_{1} \cdot \Delta L \]

Both sides of the equation contain the value \(\Delta L\). Let's divide both sides of the equation by it:

\[ \large k_{\text{parallel}} \cdot \frac{1}{2}= k_{1} \]

Let's multiply both sides of the resulting equation by the number 2:

\[ \large \boxed{ k_{\text{parallel}} = 2k_{1} } \]

The stiffness coefficient \(k_{\text{parallel}}\) of two springs connected in parallel has doubled compared to one such spring

Series connection of springs

Figure 6a illustrates a free-hanging spring. The loaded spring (Fig. 6b) is stretched to a length \(\Delta L\). Now let's take two such springs and connect them in series. Let's hang a load on these (Fig. 6c) springs.

Practice shows that a structure of two springs connected in series under the action of a load will stretch more than a single spring.

Each spring in the chain is subject to the weight of the load. Under the influence of weight, the spring stretches and transmits this weight further along the chain without changes. He stretches the next spring. And that, in turn, is stretched by the same amount \(\Delta L\).

Note: Under the influence of force, the spring stretches and transmits this tensile force further along the chain without changes

Rice. 6. A system consisting of two identical springs connected in series, more than one spring is deformed

Let's compare the stretch of two identical springs connected in series and the stretch of a single spring. In both cases, we suspend the same load weighing \(mg\) from the springs.

One spring:

\[ \large k_{1} \cdot \Delta L = m \cdot g \]

Two springs in series:

\[ \large k_{\text{last}} \cdot \Delta L \cdot 2 = m \cdot g \]

Since the right sides of the equations are the same, the left sides will also be equal:

\[ \large k_{\text{last}} \cdot \Delta L \cdot 2 = k_{1} \cdot \Delta L \]

Both sides of the equation contain the value \(\Delta L\). Let's divide both sides of the equation by it:

\[ \large k_{\text{last}} \cdot 2 = k_{1} \]

Divide both sides of the resulting equation by the number 2:

\[ \large \boxed{ k_{\text{last}} = \frac{k_{1}}{2} } \]

The stiffness coefficient \(k_{\text{last}}\) of two springs connected in series will be halved compared to one such spring

Spring connection stiffness formula

Do not forget that in some cases the body is connected by several springs. Such systems have become very widespread. Determining rigidity in this case is much more difficult. Among the features of the connection, the following points can be noted:

1. A parallel connection is characterized by the fact that the parts are placed in series. This method can significantly increase the elasticity of the created system.
2. The sequential method is characterized by the fact that the parts are connected to each other. This method of connection significantly reduces the degree of elasticity, but allows a significant increase in maximum elongation. In some cases, it is the maximum extension that is required.

Read also: What is the difference between an angle grinder and an angle grinder?

In both cases, a certain formula is used that determines the characteristics of the connection. The modulus of elastic force may vary significantly depending on the characteristics of a particular product.

When connecting products in series, the indicator is calculated as follows: 1/k=1/k1+1/k2+…+1/kn. The indicator in question is considered a rather important property, in this case it decreases. The parallel connection method is calculated as follows: k=k1+k2+…kn.

Such formulas can be used in a wide variety of calculations, most often at the time of solving mathematical problems.

Potential energy of a compressed or stretched spring

A spring compressed (the left part of Fig. 7) or stretched (the right part of Fig. 7) by a length \(\Delta L\) has the potential to return to its original state and at the same time do work, for example, to move a load. In such cases, physicists say that the spring has potential energy.

Rice. 7. A deformed - compressed or stretched spring has potential energy

This energy depends on the spring constant and on its elongation (or shortening during compression).

The greater the stiffness (elasticity) of the spring, the greater its potential energy. By increasing the elongation of the spring, we obtain an increase in its potential energy according to the quadratic law:

\[ \large \boxed{ E_{p} = \frac{k}{2} \cdot \left( \Delta L \right)^{2} }\]

\( E_{p} \left( \text{J} \right)\) – potential energy of a compressed or stretched spring;

\( \Delta L \left(\text{m} \right) \) – spring elongation;

\( \displaystyle k \left(\frac{H}{\text{m}} \right) \) – coefficient of stiffness (elasticity) of the spring.

Coil spring stiffness coefficient

In practice and in physics, it is cylindrical springs that have become quite widespread. Their key features include the following:

1. When creating, a central axis is specified, along which most of the various forces act.
2. In the production of the product in question, wire of a certain diameter is used. It is made of a special alloy or ordinary metals. Do not forget that the material must have increased elasticity.
3. The wire is wound in turns along the axis. It is worth considering that they can be the same or different diameters. The cylindrical version has become quite widespread, but the cylindrical version is characterized by greater stability; in the compressed state, the part has a small thickness.
4. The main parameters include the large, medium and small diameter of the turns, the diameter of the wire, and the pitch of the individual rings.

Do not forget that there are two types of parts: compression and tension. Their stiffness coefficient is determined by the same formula. The difference is this:

1. The compression version is characterized by a distant arrangement of turns. Due to the distance between them, compression is possible.
2. The model, designed for stretching, has rings located almost closely. This shape determines that maximum elastic force is achieved with minimal stretching.
3. There is also a design option that is designed for torsion and bending. Such a detail is calculated using certain formulas.

Calculation of the coefficient of a cylindrical spring can be carried out using the previously specified formula. It determines that the indicator depends on the following parameters:

1. Outer radius of the rings. As previously noted, when manufacturing a part, an axis is used, around which the rings are wound. At the same time, do not forget that the average and inner diameters are also distinguished. A similar indicator is indicated in the technical documentation and drawings.
2. The number of turns created. This parameter largely determines the free length of the product. In addition, the number of rings determines the stiffness coefficient and many other parameters.
3. The radius of the wire used. The starting material is wire, which is made from various alloys. In many ways, its properties influence the quality of the product in question.
4. Shear modulus, which depends on the type of material used.

The stiffness coefficient is considered one of the most important parameters, which is taken into account when carrying out a variety of calculations.

conclusions

1. Elastic bodies are those that resist deformation;
2. During deformation, a force arises in elastic bodies, it prevents deformation, it is called the elastic force;
3. Deformation is a change in the shape or size of the body;
4. There are several types of deformation: bending, torsion, shear, tension/compression;
5. The elongation of a spring is the difference between its final and initial lengths;
6. A compressed or stretched spring has potential energy (in general, any elastically deformed body has potential energy);
7. A system consisting of several identical springs will have a stiffness coefficient different from that of a single spring;
8. If the springs are connected in parallel, the stiffness coefficient of the system increases;
9. And if you connect the springs in series, the stiffness coefficient of the system will decrease.

What depends on the elasticity of the spring

Not a single mechanism or device can do without such an element. They are also used in the manufacture of furniture.

They can be found in vehicles, machine tools, door locks and handles, sofas, mattresses, watches, ballpoint pens, etc. Wherever a wire spiral is installed, its operation has a decisive influence on the functioning of the mechanism (product).

So, if it is very hard or soft, then installed in a car shock absorber will not contribute to a comfortable and safe ride on an uneven road, and if it is built into furniture, then sitting and lying on it will not be very pleasant.