The author is a professional tutor, author of textbooks for preparing for the Unified State Exam Igor Vyacheslavovich Yakovlev
Topics of the Unified State Examination codifier: forces in mechanics, elastic force, Hooke's law.
As we know, on the right side of Newton’s second law is the resultant (that is, the vector sum) of all forces applied to the body. Now we have to study the forces of interaction between bodies in mechanics. There are three types: elastic force, gravitational force and friction force. We start with elastic force.
Electromagnetic nature of elastic forces
By their physical nature, elastic forces are closer to frictional forces than to gravitational forces, since they are caused by the interaction of charged particles, which are the basis of all bodies.
However, elastic forces determine only the relative position of bodies acting on each other and appear only during deformation, while sliding friction forces arise during the relative motion of bodies.
At distances near the diameter of the molecule, the attractive forces between molecules are compensated by repulsive forces, that is, the resultant of the attractive and repulsive forces is zero. If a body is stretched, the distance between the molecules increases, and the attractive forces between the molecules become greater in magnitude than the repulsive forces. Forces appear in the body that prevent the body from stretching. When a body is compressed, the distance between the molecules decreases. The repulsive forces become greater in magnitude than the attractive forces, and this is how forces arise that resist this kind of deformation of the body.
Thus, when bodies are deformed, forces of an electromagnetic nature appear, which prevent changes in the size of the body; these are the so-called elastic forces.
Elastic force and Hooke's law: definitions
The force of elasticity is a force that prevents deformation and strives to restore the original shape and size of the body.
Examples of the action of elastic force:
- springs compress and decompress in the mattress;
- wet laundry flutters on a stretched rope;
- The archer pulls the bowstring to release an arrow.
The simplest deformations are tensile and compressive deformations.
Hooke's Law:
The deformation that occurs in an elastic body under the action of an external force is proportional to the magnitude of this force.
Coefficient k is the stiffness of the material.
There is another formulation of Hooke's law. Let us introduce the concept of relative deformation “epsilon” and material stress “sigma”:
S – cross-sectional area of the deformable body. Then Hooke's law will be written as follows: relative deformation is proportional to stress.
Here E is Young’s modulus, which depends on the properties of the material.
Hooke's law was experimentally discovered in 1660 by the Englishman Robert Hooke.
Body deformation
Definition
Body deformation is a change in the size or shape of the body. Types of deformations: tension, compression, shear, bending, torsion. Body deformations occur when some parts of the body move in relation to others.
Elastic forces arise only during deformations. The magnitude of the elastic force depends on the size of the deformation. Elastic forces are directed against the direction of displacement of parts of the body during its deformation.
For solids, there are two limiting types of deformation: elastic deformation and plastic deformation. If, after the cessation of the deforming force, the body completely restores its size and shape, then this type of deformation is called elastic. For elastic deformations, there is an unambiguous relationship between the magnitude of the deformation and the deforming force. If, after removing the deforming force, the body does not restore (or does not completely restore) its size and shape, then such deformations are called plastic.
Determination of elastic force
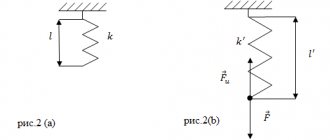
Definition The elastic force (${\overline{F}}_{upr}$) is the force that acts from the body subjected to deformation on the bodies touching it. This force is directed in the direction opposite to the displacement of body parts in a state of deformation.
Elastic forces depend on the location of bodies during their interaction and arise only when the bodies are deformed.
Elastic forces are directed perpendicular to the contact surface of the interacting bodies. The exception is shear deformation; with such deformation, the elastic force has a tangential component.
Elastic forces play an important role in mechanical equilibrium problems when a model of a non-deformable body is not sufficient.
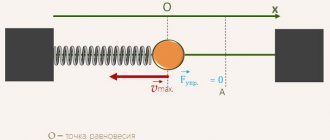
Elastic forces are a common cause of mechanical vibrations. During elastic deformation, forces appear that tend to return the body to an equilibrium position. If a body is taken out of a state of equilibrium and left to its own devices, then under the influence of elasticity there appears a movement of this body towards a position of equilibrium. As a result of the existence of inertia, the body passes through an equilibrium position and then a deformation of the opposite sign occurs, and the process is repeated.
Elastic force problems and Hooke's law with solutions
By the way! For our readers there is a 10% on any type of work .
Task No. 1. Calculation of elastic force
Condition
One end of the wire is rigidly fixed. How much force must be pulled on the other end to stretch the wire by 5 mm? The wire stiffness is known and equal to 2*10^6 N/m2.
Solution
Let's write down Hooke's law:
According to Newton's third law:
Answer: 10 kN.
Task No. 2. Finding the spring stiffness
Condition
A spring with a stiffness of 100 N/m was cut into two parts. What is the stiffness of each spring?
Solution
By definition, stiffness is inversely proportional to length. With the same force F, the uncut spring will stretch by x, and the cut spring will stretch by x1=x/2.
Answer: 200 N/m
When a spring is stretched, complex torsional and bending deformations occur in its coils, but we do not take them into account when solving problems.
Task No. 3. Finding the acceleration of a body
Condition
A body weighing 2 kg is pulled along a smooth horizontal surface with the help of a spring, which during movement is stretched by 2 cm. The stiffness of the spring is 200 N/m. Determine the acceleration with which the body is moving.
Solution
The force that is applied to the body and causes it to move can be taken as the elastic force. According to Newton's second law and Hooke's law:
Answer: 2 m/s^2.
Task No. 4. Finding the spring stiffness from a graph
Condition
The graph shows the dependence of the modulus of elastic force on the elongation of the spring. Find the spring stiffness.
Solution
Remember that stiffness is equal to the ratio of force and elongation. The presented dependence is linear. At any point on the straight line, the ratio of the ordinate F and the abscissa x gives the result 10 N/m.
Answer: k=10 N/m.
Hooke's law
Experiments show that in almost all solids, under small elastic deformations, the size of the deformation is proportional to the deforming force. This dependence was established by the English scientist R. Hooke. The law of elastic deformation bears the name of its discoverer. At large deformations, the relationship between the magnitude of the deformation and the deforming force becomes ambiguous and precisely nonlinear, elastic deformation turns into plastic.
Hooke's law states that for small elastic deformations, the magnitude of the deformation is proportional to the force causing it. Hooke's law is valid for types of elastic deformation (tension, compression, shear, torsion, bending).
For example, tensile (compressive) deformation is characterized using such a value as absolute elongation: ($\Delta l=\left|l-l_0\right|$, where $l_0$ is the length of the undeformed rod). Then Hooke's law for the elastic force is written as:
\[F_{upr}=k\Delta l\ \left(1\right).\]
where $k$ is the elasticity coefficient, $\left[k\right]=\frac{N}{m}$. The elasticity coefficient depends on the material of the body, its size and shape.
Hooke's law is satisfied with good accuracy for deformations that appear in rods made of steel, cast iron, and other solid substances, in springs.
For any elastic deformations, constants can be introduced that characterize the elastic properties of the material only and do not depend on the size of the body. For example, Young's modulus ($E$) for an isotropic body is a characteristic of elastic properties. Young's modulus is equal to the mechanical stress ($\sigma =\frac{F}{S},\ where\ F-\ $deforming force or force arising in the body during deformation; $S$ is the area), at which the relative elongation ($ \frac{\Delta l}{l_0}$) is equal to unity for elastic deformation:
\[\frac{\Delta l}{l_0}=\frac{1}{E}\sigma \left(2\right).\]
The value of Young's modulus is determined empirically.
If the deformations of the body are small, then the elastic forces can be determined by the acceleration that these forces impart to the bodies. If the body is motionless, then the modulus of the elastic force is found from the equality to zero of the vector sum of the forces that act on the body.
So, whether the deformation will be elastic or plastic depends not only on the material of the body, but also on the magnitude of the applied load. Elastic deformations are widely used, for example, in shock-absorbing devices: springs, springs, etc. Various types of cold processing of metals are based on plastic deformation: rolling, forging, etc.
EXAMPLES OF TASKS
Part 1
1. There are two absolutely elastic springs. Under the influence of the same force, the first spring lengthened by 8 cm, and the second by 4 cm. Compare the stiffness \( k_2 \) of the second spring with the stiffness \( k_1 \) of the first spring.
1) \( k_1=k_2 \) 2) \( 4k_1=k_2 \) 3) \( 2k_1=k_2 \) 4) \( k_1=2k_2 \)
2. There are two absolutely elastic springs: one with a stiffness of 200 N/m, the other with a stiffness of 400 N/m. Compare the elastic force \( F_2 \) arising in the second spring with the elastic force \( F_1 \) arising in the first spring, when their elongation is the same.
1) \( F_2=F_1 \) 2) \( F_2=4F_1 \) 3) \( 2F_2=F_1 \) 4) \( 0.5F_2=F_1 \)
3. The student, stretching the dynamometer spring sequentially by 1N, 2N, 3N and 4N, measured its elongation each time and entered the measurement results into the table. Determine the stiffness of the dynamometer spring from the table data.
1) 0.02 N/m 2) 0.5 N/m 3) 2 N/m 4) 50 N/m
4. The figure shows graphs of the dependence of elastic force on elongation. Compare the spring stiffness.
1) \( k_2=k_1 \) 2) \( k_2>k_1 \) 3) \( k_2 4) \( k_2\geq k_1 \)
5. The student performed an experiment to measure the elongation \( x \) of springs when hanging weights from them. The results obtained by the student are presented in the figure in the form of a diagram. What conclusion about the stiffness of the springs \( k_1 \) and \( k_2 \) can be drawn from the analysis of the diagram if loads of the same mass were suspended from the ends of the springs?
1) \( k_2=4k_1 \) 2) \( k_1=2k_2 \) 3) \( k_2=2k_1 \) 4) \( k_1=k_2 \)
6. Under the influence of a force of 3 N, the spring lengthens by 4 cm. What is the force under which the elongation of this spring will be 6 cm?
1) 3.5 N 2) 4 N 3) 4.5 N 4) 5 N
7. Two springs are stretched by equal forces. The stiffness of the first spring \( k_1 \) is 2 times greater than the stiffness of the second spring \( k_2 \). The elongation of the first spring \( \Delta l_1 \), the elongation of the second spring \( \Delta l_2 \) is equal to
1) \( 0.5\Delta l_1 \) 2) \( 0.67\Delta l_1 \) 3) \( 1.5\Delta l_1 \) 4) \( 2.5\Delta l_1 \)
8. There is a box in an elevator moving downward with uniform acceleration from a state of rest. Box weight module
1) equal to the gravity modulus 2) greater than the gravity modulus 3) less than the gravity modulus 4) increases with increasing elevator speed
9. A person experiences overload when
1) uniform downward movement 2) uniform upward movement 3) uniformly accelerated upward movement from a state of rest 4) uniformly accelerated downward movement with acceleration of gravity
10. The difference in body weight at the equator and at the pole can be detected
A. Weighing a body on a lever scale B. Weighing a body on a spring scale
Correct answer
1) only A 2) only B 3) both A and B 4) neither A nor B
11. Establish a correspondence between the physical quantity (left column) and the nature of its change (right column) when the dynamometer spring is stretched. In your answer, write down the numbers of the selected answers in a row.
PHYSICAL QUANTITY A. Modulus of elastic force of the spring B. Stiffness of the spring B. Modulus of elongation of the spring
NATURE OF CHANGE IN QUALITY 1) decreases 2) increases 3) does not change
12. From the statements below, choose two that are correct and write their numbers in the table.
1) Hooke's law is valid for any deformation. 2) The elastic force is directed in the direction opposite to the deformation. 3) Stiffness depends only on the material from which the body is made. 4) The weight of a body is always equal to the force of gravity acting on it. 5) Weight is applied to a support or suspension.
Part 2
13. A load weighing 5 kg begins to be lifted vertically upward with an acceleration of 2 m/s2. What is the weight of the cargo?