Physics is an incredibly fascinating science, once you figure out what's what. And the formulas in it reflect real physical processes, only in numbers. And if you understand why the formula is the way it is, then it will be much easier to learn. But it’s impossible to tell everything at once, and today we’ll figure out how to find mass through density and volume.
Before you begin to study the formulas for mass, density and volume, you should clarify some details:
- Firstly, the volume of a substance depends on temperature . When heated, a solid expands, and at low temperatures it contracts. There are also special issues, as in the case of liquid hydrogen. It cannot exist at high temperatures because it will turn into gas.
- Secondly, different organizations and countries have their own standards for the conditions under which measurements are taken . In other words, the numerical density of the same substance will differ in different countries. Therefore, before asserting that the indicators are incorrect or correct, it is necessary to clarify the conditions under which these indicators were obtained.
- Thirdly, in addition to temperature, the volume factor can also be influenced by indicators such as atmospheric pressure . It is especially important when measuring the density of gases, since it has practically no effect on solids.
The formula and the amazing history of its origin
The most common formula for most cases is: m = pV, where m is the mass of the body, p and V are the density of the substance and its volume occupied in space, respectively. You can, of course, not bother and calculate everything on online resources, but knowing the formula is still useful. Accordingly, V = m/p, p = m/V.
The most interesting thing is that the formula was found by a man who ran naked down the street and was at the same time a friend of the king. Interesting? Then the next three paragraphs are for you.
There was such a tyrant king in Ancient Greece as Hiero II. He began to suspect that his crown was not made of pure gold and that the jewelers had cheated him. But Hiero did not know how to prove this. Then he turned to the smartest man of that time - Archimedes. Having received an order to deal with matters of national importance, Archimedes day after day began to look for a solution to the issue.
Oh, and the scientist had a difficult task. After all, at that time there were neither the necessary formulas , nor modern devices, nor Google to quickly find a solution. And then one day, having come to the bathhouse and immersed himself in it, Archimedes noticed that the pouring water was equal in volume to what was immersed in the water.
Eureka! – Archimedes shouted and hurried naked to his laboratory to conduct experiments. The scientist put all the data in his head and later did the following experiment: he took the crown and lowered it into the water. Then he took a piece of gold of the same weight and lowered it into the water. The volume of displaced water turned out to be different. If the crown were made of pure gold, then its volume and the volume of the ingot would match. This proved that the jewelers had deceived the king. Who would have thought that one of the greatest discoveries came about thanks to deceivers, a tyrant and a scientist.
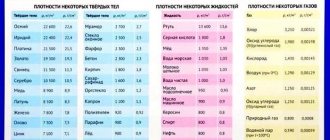
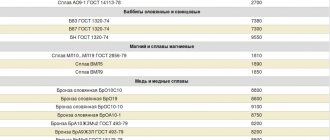
Table of densities of some substances
The density of many substances is known in advance and can be easily found using the corresponding table.
When working with it, it is important to pay attention to the dimensions and not to forget that all data was collected under normal conditions: room temperature of 20 degrees Celsius, as well as a certain pressure, air humidity, and so on.
Densities of other, rarer substances can be found online.
At least one of the density values is worth remembering, as it often appears in problems. This is the density of water - 1000 kg/m3 or 1 g/cm3.
Designations and terms
The following is a list of concepts and their definition in terms of concepts about density measurements:
- Mass is the density of a body multiplied by its volume occupied in space. This is also a quantity that determines the strength of the gravitational field on an object.
- Volume is a physical quantity that characterizes the amount of space occupied by an object.
- Density determines how much of a substance fits in a volume at a certain weight under standard conditions.
- Normal/standard conditions have different meanings in different organizations. These conditions include ambient temperature, atmospheric pressure and, in some cases, other parameters.
- Atmospheric pressure is a concept used more for gases, since it has a greater influence on their volume than on solids. Atmospheric pressure can be defined as the force exerted by the air on the Earth under the influence of the gravitational field.
- Temperature is a physical indicator of the degree of heating of a substance. The higher the temperature, the greater the volume of the body.
Formula for the dependence of mass on volume and density
To find the density of a liquid or solid, there is a basic formula: density equals mass divided by volume.
It is written like this:
And from it two more formulas can be derived.
Formula for body volume:
And also the formula for calculating mass:
As you can see, the latter is very easy to remember: this is the only formula where two units need to be multiplied.
To remember this relationship, you can use a drawing in the form of a “pyramid”, divided into three sections, at the top of which there is mass, and in the lower corners - density and volume.
Things are somewhat different with gases.
Calculating their weight is much more difficult, since gases do not have a constant density: they disperse and occupy the entire volume available to them.
For this, the concept of molar mass is useful, which can be found by adding up the mass of all atoms in the formula of a substance using data from the periodic table.
The second unit we need is the amount of substance in moles. It can be calculated using the reaction equation. You can learn more about this in the chemistry course.
Another way to find the molar amount is through the volume of gas, which must be divided by 22.4 liters. The last number is the volume constant that is worth remembering.
As a result, knowing the two previous quantities, we can determine the mass of the gas:
where M is the molar mass and n is the amount of substance.
The result will be in grams, so to solve physics problems it is important to remember to convert it to kilograms by dividing by 1000. The numbers in this formula can often be quite complex, so you may need a calculator for the calculations.
Another non-standard case that you may encounter is the need to find the density of the solution
. For this, there is a formula for average density, constructed similarly to the formulas for other average values.
For two substances it can be calculated as follows:
Also, several others can be derived from this formula, depending on which quantities are known from the conditions of the problem.
Examples of problem solving
Before proceeding with the examples, it should be understood that if the data is given in kilograms and cubic centimeters, then you need to either convert centimeters to meters, or convert kilograms to grams. Using the same principle, it is necessary to translate the remaining data - millimeters, tons, and so on.
Task 1 . Find the mass of a body consisting of a substance whose density is 2350 kg/m³ and has a volume of 20 m³. We apply the standard formula and easily find the value. m = p*V= 2,350 * 20 = 47,000 kg.
Task 2 . It is already known that the density of pure gold without impurities is 19.32 g/cm³. Find the mass of a precious gold chain if the volume is 3.7 cm³. Let's use the formula and substitute the values. p = m / V = 19.32/3.7 = 5.22162162 g.
Task 3 . Metal with a density of 9250 kg/m³ was delivered to the warehouse. Weight is 1,420 tons. We need to find the volume occupied by the metal. Here you first need to convert either tons to kilograms, or meters to kilometers. It will be easier to use the first method. V = m/p = 1420/9250 = 0.153513514 m³.
Weight of solid part
Home > Mass calculations > Solid part mass
05/09/2013 // Vladimir Trunov
This strange title of the article is explained only by the fact that parts of the same shape can be either solid or hollow (i.e. the next article will be called “Mass of a hollow part”).
Here is the time to remember that the mass of a body is its volume, multiplied by the density of its material (see density tables): The volume of a solid part is... its volume and nothing more.
Note : In the formulas below, all dimensions are measured in millimeters, and density is measured in grams per cubic centimeter. The letter indicates the ratio of the circumference of a circle to its diameter, which is approximately 3.14 .
Let's look at several simple forms (more complex ones, as you remember, can be made by adding or subtracting simple ones).
Mass of a parallelepiped (bar)
Volume of a parallelepiped: , where is the length, is the width, is the height. Then the mass:
Cylinder weight
Volume of the cylinder: , where is the diameter of the base, is the height of the cylinder. Then the mass:
Ball mass
Volume of the ball: , where is the diameter of the ball. Then the mass:
Ball segment mass
Volume of a ball segment: , where is the diameter of the base of the segment, and is the height of the segment. Then the mass:
Cone mass
The volume of any cone: , where is the area of the base, and is the height of the cone. For a round cone: , where is the diameter of the base, and is the height of the cone. Mass of round cone:
Mass of a truncated cone
Since it is impossible to embrace the immensity, we will consider only a round truncated cone. Its volume is the difference between the volumes of two nested cones: with bases and : , where , . After algebraic transformations that are not interesting to anyone, we get: , where is the diameter of the larger base, is the diameter of the smaller base, is the height of the truncated cone. Hence the mass:
Mass of the pyramid
The volume of any pyramid is equal to one third of the product of the area of its base and its height (the same as for cones (we often don’t notice how favorable the universe is to us)): , where is the area of the base and is the height of the pyramid. For a pyramid with a rectangular base: , where is the width, is the length, is the height of the pyramid. Then the mass of the pyramid is:
Mass of a truncated pyramid
Consider a truncated pyramid with a rectangular base. Its volume is the difference between the volumes of two similar pyramids with bases and: , where , . Having crossed out half of the notebook sheet, we get: , where , is the width and length of the larger base, , is the width and length of the smaller base, and is the height of the pyramid. And, leaving the remaining half of the sheet alone, based on symmetry considerations alone, we can write another formula, which differs from the previous one only by replacing W with L and vice versa. What is the difference between length and width? Only that we called them that. Let's call it the other way around and get: . Then the mass of a truncated rectangular pyramid is:
or
For a pyramid with a square base (, ), the formula looks simpler:
mass calculation
Weight of the wedding ring
Weight of hollow part
Introduction to Mass Calculations
Related Posts
Why and who needs to know these formulas
Every country has standards by which products are manufactured. It doesn’t matter what industry it is - food, chemical or other. Standards can also be global. So, in order for the products produced at factories to meet these standards, knowledge about density, mass and volume is needed.
But why would anyone adhere to someone else's rules? To begin with, these rules were not pulled out of thin air. Various businessmen from all over the world came to this and found the optimal solution that satisfies both manufacturers and end users of the product. If everyone produced products as they pleased, it would be very difficult for people to choose a manufacturer. After all, even now, with all the standards and GOSTs, the choice is simply huge.
In addition, by ignoring physics and mathematics , you can develop products at a loss or make products that will not live up to expectations and will not look the way the manufacturer intended. There are other situations where knowledge of this kind is needed - when calculating the planned volume that products will occupy in a warehouse; weight of products that will need to be transferred, etc.
This knowledge may be required by engineers, technologists, designers and other professions whose activities are related to physical materials. Of course, this knowledge may not be useful for the average person. However, it is worth remembering the incident with Archimedes and then you will understand that knowledge is protection from deception and real power!
Formula for the dependence of mass on volume and density
To find the density of a liquid or solid, there is a basic formula: density equals mass divided by volume.
It is written like this:
And from it two more formulas can be derived.
Formula for body volume:
And also the formula for calculating mass:
As you can see, the latter is very easy to remember: this is the only formula where two units need to be multiplied.
To remember this relationship, you can use a drawing in the form of a “pyramid”, divided into three sections, at the top of which there is mass, and in the lower corners - density and volume.
Things are somewhat different with gases.
Calculating their weight is much more difficult, since gases do not have a constant density: they disperse and occupy the entire volume available to them.
For this, the concept of molar mass is useful, which can be found by adding up the mass of all atoms in the formula of a substance using data from the periodic table.
The second unit we need is the amount of substance in moles. It can be calculated using the reaction equation. You can learn more about this in the chemistry course.
Another way to find the molar amount is through the volume of gas, which must be divided by 22.4 liters. The last number is the volume constant that is worth remembering.
As a result, knowing the two previous quantities, we can determine the mass of the gas:
where M is the molar mass and n is the amount of substance.
The result will be in grams, so to solve physics problems it is important to remember to convert it to kilograms by dividing by 1000. The numbers in this formula can often be quite complex, so you may need a calculator for the calculations.
Another non-standard case that you may encounter is the need to find the density of the solution
. For this, there is a formula for average density, constructed similarly to the formulas for other average values.
For two substances it can be calculated as follows:
Also, several others can be derived from this formula, depending on which quantities are known from the conditions of the problem.
Calculation of body volume based on its density
In a similar way, we express volume from the density formula:
$$V = \frac{m}{\rho}$$
To calculate the volume of a body, if its mass and density are known, you need to divide the mass by the density.
This formula for determining volume is often used in cases where bodies have a complex irregular shape.
Let's consider an example of a volume calculation problem. Milk in a bottle has a mass of 1.03 kg. Calculate the volume of the bottle.
In Table 2 of the previous paragraph we find milk: its density is $1030 \frac{kg}{m^3}$.
Given: $\rho = 1030 \frac{kg}{m^3}$ $m = 1.03 kg$
Find: $V -?$
Solution: $V = \frac{m}{\rho}$ $V = \frac{1.03 kg}{1030 \frac{kg}{m^3}} = 0.001 m^3 = 1 l$
Answer: $V = 0.001 m^3 = 1 l$.
Table of densities of some substances
The density of many substances is known in advance and can be easily found using the corresponding table.
When working with it, it is important to pay attention to the dimensions and not to forget that all data was collected under normal conditions: room temperature of 20 degrees Celsius, as well as a certain pressure, air humidity, and so on.
Densities of other, rarer substances can be found online.
At least one of the density values is worth remembering, as it often appears in problems. This is the density of water - 1000 kg/m3 or 1 g/cm3.
How to find mass by size?
Mass
bodies can be calculated using the formula m=ρ*V, where V is the volume of the body, ρ=7.87 g/cm^3 is the density of iron.
Then we determine the mass
of the sheet: m=7.87*1400=11018 g = 11.018 kg.
Interesting materials:
What should the pH of saliva be? What should the pH be in a swimming pool? How much should the opening be for the stairs to the second floor? How long should the first layer of putty dry? How long should harvested garlic dry? How much should the ceiling height in an apartment be? How high should a school table be? How long should a Phytolamp burn? How long should potatoes last after digging? How much should a leg of lamb weigh?
What is the volumetric weight of transported goods?
As is known, physical density is different for solid and liquid substances. In this regard, goods that have the same weight but different volumes may take up different space in the vehicle body. Distilled water is considered the reference unit: 1 cubic meter is equal to the weight of 1 ton.
In sea transportation, first of all, the volume of transported cargo is taken into account. In air transportation, on the contrary, the weight of the transported products is important. As for road transport, both indicators must be taken into account.
In international cargo transportation, you can often come across such a term as “Stowage Factor”, which translates as stowage factor.
According to this criterion, all cargo can be divided into several groups:
- volumetric (coefficient 1.3-2 units, with a ton of weight taking up no more than 2 cubic meters of volume);
- heavy (with a coefficient of less than 1);
- deadweight (coefficient about 1).
According to this classification, the volumetric weight of grain (wheat) has a coefficient of 1.66 (1 cubic meter with a weight of 660 kg) is classified as bulk cargo, while iron ore, which has a coefficient of 0.33, is classified as heavy cargo.
When calculating the location of containers in which products are transported, sometimes such an indicator as “loading meter” is taken into account. Since the width of a heavy load does not exceed 2.4 m, the above term refers to a meter of length of a heavy load, the area of which is within 2.4 sq.m.
How to count a large number of small objects
Sometimes you need to quickly count a large number of small items, such as screws or paper clips in a package. How to do it quickly, easily and conveniently
We count by weight
The easiest way to count small objects is with a scale. We count out one dozen objects, weigh the ten - this is how we get the weight of one dozen objects. After this, you need to weigh the complete package of items. By dividing the total mass by the mass of one ten, you can find the number of tens of items in the package.
For example, a dozen items weigh 52 grams, and the total weight of the package is 754 grams. If 754 is divided by 52, we get 14.5. This means there are 14 and a half dozen items in the package, that is, 145 pieces.
We count without scales, one by one
But what to do if you don’t have scales at hand? How to count the number of items in this case?
If there are not very many items, then this is not too difficult. Every adult can count up to two or three hundred.
But what to do if there are a lot of items and time is running out?
In this case, one simple method will help. The idea is to count out ten items at a time, but only transfer nine items from the package into a suitable container. And put every tenth one separately, it doesn’t matter - in a separate box or just on the table, the main thing is - separately.
When all the items are removed from the package, it remains to count every tenth item that is lying separately. How many tenths of items - so many tens of items in the package. This way you can easily count several hundred items.
For example, all the items were counted, and there were 54 items put aside in tenths, and there were 8 more in the package. This means that 54 tens of items is 540. To 540 we add another 8 items remaining in the package, and we get the total number - 548.
If there are more than a thousand items, you can arrange the tenth items in nines, and put the tenths separately. As a result of such a double counting circle, every tenth item will already mean hundreds. This way you can count even several thousand items.
For example, all items from a package have been counted, and there are 7 items left in the package. There were a lot of items that were put aside in tenths. Now you need to count these tenths of items using the same system as single items. We put nine in the package, and leave the tenth separately. And there were 32 hundreds of such items left separately, and there were 5 more left.
Now we count the total number. 32x100=3200, multiply the remainder of the tenth items by 10 (5x10=50) and get 50. There is still a remainder in the package - 7 items. We add everything up (3200+50+7) and get the total number of items – 3257.
By using this simple method, you can count the number of items with great accuracy, even if you don’t have a pencil or scale at hand.