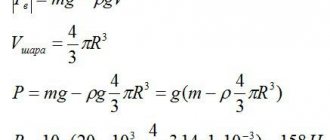From the chemistry and physics course, I remember solving problems using different indicators.
The surrounding bodies consist of substances, the mass of each depends on the size, volume and other criteria.
The density of a substance shows the numerical expression of the mass of a body in a certain volume.
There are different types of scalar physical quantity.
Experienced confirmation
Consider the experiment presented in Figure 1.
Figure 1. Weighing two identical bodies consisting of different substances.
Let's take two identical cylinders: they have the same shape and volume, but are made of different materials.
One is made of aluminum and the other is made of lead. Let's place them on different scales.
As a result, we will see that the mass of an aluminum cylinder will be almost 4 times less than the mass of a lead cylinder.
Bodies that have equal volumes, but consist of different substances, have different masses.
The figure shows 3 bodies weighing 100 g: ice, iron and gold.
Figure 2. Bodies of the same mass, but consisting of different substances.
Here are bodies of the same mass, but look at their volume. The volume of ice will be almost 8.5 times greater than the volume of a piece of iron of the same mass. And the volume of gold will be almost 3 times less than the volume of iron.
Bodies with equal masses, but consisting of different substances, have different volumes.
Formula for finding density [edit | edit code]
Density (density of a homogeneous body or average density of a non-uniform one) is found by the formula:
ρ = m V , ho =>,>
where m
— body weight,
V
— its volume; the formula is simply a mathematical notation of the definition of the term "density" given above.
- When calculating the density of gases under normal conditions, this formula can also be written in the form:
ρ = MV m , ho =>>,>where M
is the molar mass of the gas, V m > is the molar volume (under normal conditions approximately equal to 22.4 l/mol).
The body density at a point is written as
ρ = dmd V , ho =>,>
then the mass of an inhomogeneous body (a body with a density depending on the coordinates) is calculated as
m = ∫ ρ ( r ) d 3 r = ∫ ρ ( r ) d V = ∫ dm . ho (mathbf )d^<3>mathbf=int ho (mathbf)dV=int dm.>
Determination of the density of a substance
The above properties of the substances that make up bodies are explained by the fact that different substances have different densities.
Let's consider two bodies with a volume of $1 m^3$ each. If they consist of different substances, then their masses will also be different.
So, aluminum of this volume will have a mass of 2700 kg, and lead of the same volume ($1 m^3$) will have a mass of 11,300 kg.
Figure 3 shows other examples of bodies of equal volume, but consisting of different substances.
Figure 3. Bodies of equal volume, consisting of different substances.
Density shows the mass of a substance taken in a volume of $1 m^3$ (or $1 cm^3$). To find the density of a substance, you need to divide the mass of a body by its volume.
Let's give a definition:
Density is a physical quantity that is equal to the ratio of the mass of a body to its volume:
$$density = \frac{mass}{volume}$$
or
$$\rho = \frac{m}{V}$$
where $\rho$ (“po”) is the density of the substance, $m$ is the mass of the body, $V$ is the volume of the body.
general characteristics
Each element occupies an individual value. The definition of density may be denoted by the Greek letter ρ, D, or d. If the volumes of two bodies are the same, but the masses are different, then the densities are not identical.
Basic Concepts
The definitions and characteristics of the indicator have been known since the 7th grade of the school chemistry curriculum. Density is a physical quantity about the properties of a substance. This is the specific gravity of any element. There is an average and relative density. The last classification is the ratio of the density (P) of the substance to the P of the reference substance. Distilled water is often taken as the standard. The unit of measurement is P - kg/m3 in the international system.
Formula for finding density:
P = m/V
Designations:
- m is mass.
- V—volume.
In addition to the standard density formula used for solid states of substances, there is a formula for gaseous elements under normal conditions.
ρ (gas) = M/Vm M
Explanation:
- M is the molar mass of the gas [g/mol].
- Vm is the volume of gas (normally 22.4 l/mol).
For granular and porous bodies, a distinction is made between true density, calculated without taking into account voids, and specific density, calculated as the ratio of the mass of the substance to the entire volume. True P is obtained through the porosity coefficient - the proportion of void volume in the occupied volume. For bulk solids, the specific P is called bulk.
The medium between galaxies has low P values (1033 kg/m3).
Measurement methods:
- Pycnometer. Measures true P.
- Hydrometer, densimeter, density meter. Used for liquid state.
- Burik. Measures soil P.
Substances consist of molecular structures; body mass is formed from an accumulation of molecules. Similarly, the weight of a bag of caramel is the sum of the masses of all the candies in the bag. If all the sweets are the same, then the mass of the package is determined by multiplying the weight of one candy by the number of pieces.
The molecular particles of a pure substance are identical, so the weight of a drop of water is equal to the product of the mass of 1 H2O molecule by the number of constituent molecules in the drop. The density of a substance shows the mass of one cubic meter.
The density of water is 1000 kg/m³, and the mass of 1 m³ of H2O is equal to 1000 kilograms. This number can be calculated by multiplying the mass of 1 water molecule by the number of molecular particles contained in 1 m3 of volume.
The weight of ice is 900 kg/m³, which means that the weight of a cubic meter of ice is 900 kg. The unit of measurement for density is g/cm3.
If the physical masses of two bodies are equal, their volumes differ. For example, the volume of ice is nine times the volume of a block of metal alloy. The body mass is distributed unequally, sets P at each point of the body.
Influence of factors
P depends on pressure and temperature. At high pressure, the molecules adhere tightly to each other, so the substance has a significant density.
The dependence of the indicators is taken into account when calculating P. As the temperature rises, P decreases due to thermal expansion, during which the volume increases but the mass remains the same. If the temperature decreases, P increases, although there are substances whose P behaves differently under certain temperature conditions. These are water, bronze, cast iron. During a phase transition, modification of the state of aggregation, P changes abruptly. The calculation conditions depend on the properties of substances and molecular elements. For different natural objects, P varies over a wide range.
The P of water is lower than the P of ice due to the molecular structure of the solid form of the liquid. A substance, passing from liquid to solid form, changes its molecular structure, the distance between its constituent particles narrows and density increases. In winter, if you forget to drain the water from the pipes, they burst into pieces after freezing. P H2O is affected by impurities. Sea water has a higher P sign than fresh water. When two types of liquid are combined in one glass, the fresh liquid will remain on the surface. The higher the salt concentration, the more water there is.
When the density of a substance is greater than P water, it will be completely immersed in water. Objects made from low-P material will float on the surface of the water. In practice, these properties are used by humans. When constructing ships, design engineers use materials with high P. Ships, motor ships, and yachts can sink while sailing; special cavities filled with air are created in the hulls of ships, because its P is lower than the density of water.
In order for fishing bait to sink into the water, it is burdened with a heavy-density material, for example, a weight made of metal (usually lead). The density of the alloy is higher than that of H2O.
Greasey stains of oil, petroleum, and gasoline remain on the surface of the water due to the low P of oily substances.
Density units
In SI, the density of a substance is measured in kilograms per cubic meter ($1 \frac{kg}{m^3}$).
Another unit of measurement is also often used - grams per cubic centimeter ($1 \frac{g}{cm^3}$) (Figure 4).
Figure 4. Densities of various substances in $\frac{g}{cm^3}$.
Sometimes we need to convert the density of substances expressed in $\frac{kg}{m^3}$ into $\frac{g}{cm^3}$.
Let's express the density of marble ($2700 \frac{kg}{m^3}$) in $\frac{g}{cm^3}$:
$$\rho = 2700 \cdot \frac{1 kg}{1 m^3} = 2700 \cdot \frac{1000 g}{1,000,000 cm^3} = \frac{2700}{1000} \cdot \ frac{g}{cm^3} = 2.7 \frac{g}{cm^3}$$
Dependence of density on temperature [edit | edit code ]
As a rule, as the temperature decreases, the density increases, although there are substances whose density behaves differently in a certain temperature range, for example, water, bronze and cast iron. Thus, the density of water has a maximum value at 4 °C and decreases both with increasing and decreasing temperature relative to this value.
When the state of aggregation changes, the density of a substance changes abruptly: the density increases during the transition from a gaseous state to a liquid and when the liquid solidifies. Water, silicon, bismuth and some other substances are exceptions to this rule, since their density decreases when solidified.
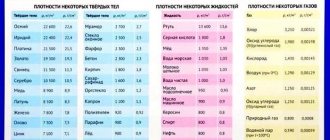
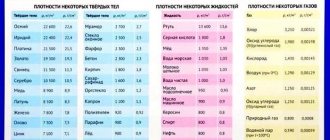
Densities of various solids
| Solid | $\rho, \frac{kg}{m^3}$ | $\rho, \frac{g}{cm^3}$ | Solid | $\rho, \frac{kg}{m^3}$ | $\rho, \frac{g}{cm^3}$ |
| Osmium | 22 600 | 22,6 | Marble | 2700 | 2,7 |
| Iridium | 22 400 | 22,4 | Glass | 2500 | 2,5 |
| Platinum | 21 500 | 21,5 | Porcelain | 2300 | 2,3 |
| Gold | 19 300 | 19,3 | Concrete | 2300 | 2,3 |
| Lead | 11 300 | 11,3 | Brick | 1800 | 1,8 |
| Silver | 10 500 | 10,5 | Sugar | 1600 | 1,6 |
| Copper | 8900 | 8,9 | Plexiglas | 1200 | 1,2 |
| Brass | 8500 | 8,5 | Capron | 1100 | 1,1 |
| Steel, iron | 7800 | 7,8 | Polyethylene | 920 | 0,92 |
| Tin | 7300 | 7,3 | Paraffin | 900 | 0,90 |
| Zinc | 7100 | 7,1 | Ice | 900 | 0,90 |
| Cast iron | 7000 | 7,0 | Dry oak | 700 | 0,70 |
| Corundum | 4000 | 4,0 | Dry pine | 400 | 0,40 |
| Aluminum | 2700 | 2,7 | Cork | 240 | 0,24 |
Table 1
Densities of astronomical objects [edit | edit code ]
- For the average densities of the celestial bodies of the Solar System, see the inset.
- The interplanetary medium in the Solar System is quite heterogeneous and can change over time, its density in the vicinity of the Earth
10 −21 ÷10 −20 kg/m³. Density of the interstellar medium
10 −23 ÷10 −21 kg/m³.
- The density of the intergalactic medium is 2×10 −34 ÷5×10 −34 kg/m³.
- The average density of red giants is many orders of magnitude lower due to the fact that their radius is hundreds of times larger than that of the Sun.
- Density of white dwarfs 10 8 ÷10 12 kg/m³
- The density of neutron stars is of the order of 10 17 ÷10 18 kg/m³.
- The average (by volume under the event horizon) density of a black hole depends on its mass and is expressed by the formula:
ρ = 3 c 6 32 π M 2 G 3 . ho =<3,c^<6>><32pi M^<2>G^<3>>>.>The average density falls in inverse proportion to the square of the black hole mass (ρ
M −2 ). So, if a black hole with a mass on the order of the solar mass has a density of about 10 19 kg/m³, exceeding the nuclear density (2 × 10 17 kg/m³), then a supermassive black hole with a mass of 10 9 solar masses (the existence of such black holes is assumed in quasars ) has an average density of about 20 kg/m³, which is significantly less than the density of water (1000 kg/m³).
Densities of various liquids
| Liquid | $\rho, \frac{kg}{m^3}$ | $\rho, \frac{g}{cm^3}$ | Liquid | $\rho, \frac{kg}{m^3}$ | $\rho, \frac{g}{cm^3}$ |
| Mercury | 13 600 | 13,60 | Kerosene | 800 | 0,80 |
| Sulfuric acid | 1800 | 1,80 | Alcohol | 800 | 0,80 |
| Honey | 1350 | 1,35 | Oil | 800 | 0,80 |
| Sea water | 1030 | 1,03 | Acetone | 790 | 0,79 |
| Whole milk | 1030 | 1,03 | Ether | 710 | 0,41 |
| The water is clean | 1000 | 1,00 | Petrol | 710 | 0,71 |
| Sunflower oil | 930 | 0,93 | Liquid Tin (at $400^{\circ}$) | 6800 | 6,80 |
| Machine oil | 900 | 0,90 | Liquid air (at $-194^{\circ}$) | 860 | 0,86 |
table 2
Density of some types of wood [ edit | edit code ]
Wood density, g/cm³
| Balsa | 0,15 | Siberian fir | 0,39 |
| Sequoia evergreen | 0,41 | Spruce | 0,45 |
| Willow | 0,46 | Alder | 0,49 |
| Aspen | 0,51 | Pine | 0,52 |
| Linden | 0,53 | horse chestnut | 0,56 |
| Edible chestnut | 0,59 | Cypress | 0,60 |
| Bird cherry | 0,61 | Hazel | 0,63 |
| Walnut | 0,64 | Birch | 0,65 |
| Cherry | 0,66 | Smooth elm | 0,66 |
| Larch | 0,66 | Field maple | 0,67 |
| Teak | 0,67 | Beech | 0,68 |
| Pear | 0,69 | Oak | 0,69 |
| Switenia (Mahogany) | 0,70 | Sycamore | 0,70 |
| Zhoster (buckthorn) | 0,71 | Yew | 0,75 |
| Ash | 0,75 | Plum | 0,80 |
| Lilac | 0,80 | Hawthorn | 0,80 |
| Pecan (cariah) | 0,83 | Sandalwood | 0,90 |
| Boxwood | 0,96 | Ebony | 1,08 |
| Quebracho | 1,21 | Lignum vitae | 1,28 |
| Cork | 0,20 |
Densities of various gases
| Gas | $\rho, \frac{kg}{m^3}$ | $\rho, \frac{g}{cm^3}$ | Gas | $\rho, \frac{kg}{m^3}$ | $\rho, \frac{g}{cm^3}$ |
| Chlorine | 3,210 | 0,00321 | Carbon monoxide | 1,250 | 0,00125 |
| Carbon dioxide | 1,980 | 0,00198 | Natural gas | 0,800 | 0,0008 |
| Oxygen | 1,430 | 0,00143 | Water vapor (at $100^{\circ}$) | 0,590 | 0,00059 |
| Air (at $0^{\circ}C$ | 1,290 | 0,00129 | Helium | 0,180 | 0,00018 |
| Nitrogen | 1,250 | 0,00125 | Hydrogen | 0,090 | 0,00009 |
Table 3
Range of densities in nature [ edit | edit code ]
For various natural objects, density varies over a very wide range.
- The intergalactic medium has the lowest density (2·10−31 -5·10−31 kg/m³, excluding dark matter) [3].
- The density of the interstellar medium is approximately 10 −23 —10 −21 kg/m³.
- The average density of red giants within their photospheres is much less than that of the Sun - due to the fact that their radius is hundreds of times larger for a comparable mass.
- The density of hydrogen gas (the lightest gas) under normal conditions is 0.0899 kg/m³.
- The density of dry air under normal conditions is 1.293 kg/m³.
- One of the heaviest gases, tungsten hexafluoride, is approximately 10 times heavier than air (12.9 kg/m³ at +20 °C)
- Liquid hydrogen at atmospheric pressure and a temperature of −253 °C has a density of 70 kg/m³.
- The density of liquid helium at atmospheric pressure is 130 kg/m³.
- The average density of the human body is from 940-990 kg/m³ with a full inhalation, to 1010-1070 kg/m³ with a full exhalation.
- The density of fresh water at 4 °C is 1000 kg/m³.
- The average density of the Sun within the photosphere is about 1410 kg/m³, approximately 1.4 times higher than the density of water.
- Granite has a density of 2600 kg/m³.
- The average density of the Earth is 5520 kg/m³.
- The density of iron is 7874 kg/m³.
- The density of metallic uranium is 19100 kg/m³.
- The density of atomic nuclei is approximately 2·10 17 kg/m³.
- Theoretically, the upper limit of density according to modern physical concepts is the Planck density of 5.1⋅10 96 kg/m³.