Physics is an incredibly fascinating science, once you figure out what's what. And the formulas in it reflect real physical processes, only in numbers. And if you understand why the formula is the way it is, then it will be much easier to learn. But it’s impossible to tell everything at once, and today we’ll figure out how to find mass through density and volume.
Before you begin to study the formulas for mass, density and volume, you should clarify some details:
- Firstly, the volume of a substance depends on temperature . When heated, a solid expands, and at low temperatures it contracts. There are also special issues, as in the case of liquid hydrogen. It cannot exist at high temperatures because it will turn into gas.
- Secondly, different organizations and countries have their own standards for the conditions under which measurements are taken . In other words, the numerical density of the same substance will differ in different countries. Therefore, before asserting that the indicators are incorrect or correct, it is necessary to clarify the conditions under which these indicators were obtained.
- Thirdly, in addition to temperature, the volume factor can also be influenced by indicators such as atmospheric pressure . It is especially important when measuring the density of gases, since it has practically no effect on solids.
The formula and the amazing history of its origin
The most common formula for most cases is: m = pV, where m is the mass of the body, p and V are the density of the substance and its volume occupied in space, respectively. You can, of course, not bother and calculate everything on online resources, but knowing the formula is still useful. Accordingly, V = m/p, p = m/V.
The most interesting thing is that the formula was found by a man who ran naked down the street and was at the same time a friend of the king. Interesting? Then the next three paragraphs are for you.
There was such a tyrant king in Ancient Greece as Hiero II. He began to suspect that his crown was not made of pure gold and that the jewelers had cheated him. But Hiero did not know how to prove this. Then he turned to the smartest man of that time - Archimedes. Having received an order to deal with matters of national importance, Archimedes day after day began to look for a solution to the issue.
Oh, and the scientist had a difficult task. After all, at that time there were neither the necessary formulas , nor modern devices, nor Google to quickly find a solution. And then one day, having come to the bathhouse and immersed himself in it, Archimedes noticed that the pouring water was equal in volume to what was immersed in the water.
Eureka! – Archimedes shouted and hurried naked to his laboratory to conduct experiments. The scientist put all the data in his head and later did the following experiment: he took the crown and lowered it into the water. Then he took a piece of gold of the same weight and lowered it into the water. The volume of displaced water turned out to be different. If the crown were made of pure gold, then its volume and the volume of the ingot would match. This proved that the jewelers had deceived the king. Who would have thought that one of the greatest discoveries came about thanks to deceivers, a tyrant and a scientist.
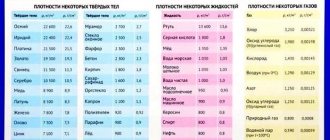
Table of densities of some substances
The density of many substances is known in advance and can be easily found using the corresponding table.
When working with it, it is important to pay attention to the dimensions and not to forget that all data was collected under normal conditions: room temperature of 20 degrees Celsius, as well as a certain pressure, air humidity, and so on.
Densities of other, rarer substances can be found online.
At least one of the density values is worth remembering, as it often appears in problems. This is the density of water - 1000 kg/m3 or 1 g/cm3.
Designations and terms
The following is a list of concepts and their definition in terms of concepts about density measurements:
- Mass is the density of a body multiplied by its volume occupied in space. This is also a quantity that determines the strength of the gravitational field on an object.
- Volume is a physical quantity that characterizes the amount of space occupied by an object.
- Density determines how much of a substance fits in a volume at a certain weight under standard conditions.
- Normal/standard conditions have different meanings in different organizations. These conditions include ambient temperature, atmospheric pressure and, in some cases, other parameters.
- Atmospheric pressure is a concept used more for gases, since it has a greater influence on their volume than on solids. Atmospheric pressure can be defined as the force exerted by the air on the Earth under the influence of the gravitational field.
- Temperature is a physical indicator of the degree of heating of a substance. The higher the temperature, the greater the volume of the body.
Peculiarities
In the case of porous or granular substances (the former includes, for example, shell rock, the latter – cereals from which porridge is prepared), two densities are distinguished:
- true - minus voids filled with air or liquid;
- bulk - determined by the method described above, when the volume of porous/bulk material includes voids.
The real density is calculated from the apparent (bulk) density through a coefficient determined in practice - excluding voids.
As temperature increases, the density of a substance decreases, although there are exceptions, for example, water. At 4 °C it is most dense; with cooling and heating, the value decreases, and ice is lighter than liquid water.
Examples of problem solving
Before proceeding with the examples, it should be understood that if the data is given in kilograms and cubic centimeters, then you need to either convert centimeters to meters, or convert kilograms to grams. Using the same principle, it is necessary to translate the remaining data - millimeters, tons, and so on.
Task 1 . Find the mass of a body consisting of a substance whose density is 2350 kg/m³ and has a volume of 20 m³. We apply the standard formula and easily find the value. m = p*V= 2,350 * 20 = 47,000 kg.
Task 2 . It is already known that the density of pure gold without impurities is 19.32 g/cm³. Find the mass of a precious gold chain if the volume is 3.7 cm³. Let's use the formula and substitute the values. p = m / V = 19.32/3.7 = 5.22162162 g.
Task 3 . Metal with a density of 9250 kg/m³ was delivered to the warehouse. Weight is 1,420 tons. We need to find the volume occupied by the metal. Here you first need to convert either tons to kilograms, or meters to kilometers. It will be easier to use the first method. V = m/p = 1420/9250 = 0.153513514 m³.
How to find the mass of a solute formula?
Let us calculate the mass of the dissolved substance using the formula: m (в − ва) = w (в − ва) ⋅ m (р − ра); m (H 2 SO 4 ) = w ( H 2 SO 4 ) ⋅ m ( p − ra ) = 0.64 ⋅ 200 = 128 g.
Interesting materials:
How long does it take to charge Mi Band 4? How long does it take to charge i12 Tws? How much is a midshipman's salary? How much does an operative earn? How long does it take to receive child benefit? How long does it take to receive an extract from the Unified State Register through the MFC? How long do dumplings live? How long do long-lived crows live? How many lives did the First World War take? How many zones does the root have?
Why and who needs to know these formulas
Every country has standards by which products are manufactured. It doesn’t matter what industry it is - food, chemical or other. Standards can also be global. So, in order for the products produced at factories to meet these standards, knowledge about density, mass and volume is needed.
But why would anyone adhere to someone else's rules? To begin with, these rules were not pulled out of thin air. Various businessmen from all over the world came to this and found the optimal solution that satisfies both manufacturers and end users of the product. If everyone produced products as they pleased, it would be very difficult for people to choose a manufacturer. After all, even now, with all the standards and GOSTs, the choice is simply huge.
In addition, by ignoring physics and mathematics , you can develop products at a loss or make products that will not live up to expectations and will not look the way the manufacturer intended. There are other situations where knowledge of this kind is needed - when calculating the planned volume that products will occupy in a warehouse; weight of products that will need to be transferred, etc.
This knowledge may be required by engineers, technologists, designers and other professions whose activities are related to physical materials. Of course, this knowledge may not be useful for the average person. However, it is worth remembering the incident with Archimedes and then you will understand that knowledge is protection from deception and real power!
General methods for determining planetary masses
The most classic way to find out the mass of planets is by calculation using the formulas of Kepler’s third law. It states that the squares of the orbital periods of the planets are related in the same way as the cubes of the semi-major axes of the orbits. Newton clarified this law a little by introducing the masses of celestial bodies into the formula. The output is the following formula:
In this way, you can find the mass of all the planets of the Solar System and the Sun itself. Both the periods of revolution and the semi-major axes of the orbits of the planets of the Solar System are easily measured by astronomical techniques, accessible even without complex instruments. And since we have already calculated the mass of the Earth, we can substitute all the numbers into the formula and find the final result.
In relation to exoplanets and other stars (but only double ones), astronomy usually uses the method of analyzing visible disturbances and oscillations. It is based on the fact that all massive bodies “perturb” each other’s orbits.
Such calculations were used to discover the planets Neptune and Pluto, even before their visual detection, as they say “at the tip of a pen.”
But you can find out the exact weight of such products much easier on our website.
We bring to your attention a universal interactive mass calculator for independently calculating the mass of products of various shapes from cylindrical or sheet materials. Its peculiarity is that it allows you to find out the weight of a part or product not only made of rolled metal and alloys, but also any other materials: wood and MDF, plastics and polymers, paper, cardboard, rubber, concrete, brick. This can be done simply by entering the overall dimensions of the part, minus the sizes of holes and slots, as well as the density coefficient of the material from which the part is made. The exact data can be found in the adjacent table.
The mass of a cylindrical part is calculated as follows:
• In the appropriate fields of the mass calculator, enter the dimensional indicators: diameter, length and reference density of the material - the calculator will calculate the total mass of the product. • The second step - if the product has protrusions or steps - you need to add their dimensions. • And the third step is to subtract the dimensions of the holes, recesses, slits. • The result is the exact calculated mass of the cylindrical part.
The mass of a sheet part is calculated as follows:
• In the appropriate fields of the mass calculator, enter the dimensional indicators: width, length, thickness and reference density of the material - the calculator will calculate the total mass of the product. • The second step - if the product has protrusions - you need to add their dimensions. • And the third step is to subtract the dimensions of the rectangular or round holes. • The result is the exact calculated mass of the sheet metal part.
Our product weight calculator will be useful both for the designer and for customers, because it allows you to very quickly and with almost 100% accuracy obtain the necessary data regarding the weight of the product without complex mathematical calculations and weighing procedures.
Please note that the default weight in the calculator is steel grade 40 GOST 1050-88.
Source
Mass values of the planets of the solar system
So, we have figured out the general methods for calculating the masses of various celestial bodies and calculated the values for the Moon, Earth and Galaxy. Let's now rank the planets of our system by their mass.
The ranking with the largest mass of planets in the Solar System is headed by Jupiter, which was one order short of being enough for our system to become a binary system. A little more and we could have two Suns, the second instead of Jupiter. So, the mass of this gas giant is 1.9 × 10²⁷ kg.
It is interesting that Jupiter is the only planet in our system whose center of mass of rotation with the Sun is located outside the surface of the star. It is located approximately 7% of the distance between them from the surface of the Sun.
The second most massive planet is Saturn, its mass is 5.7 × 10²⁶ kg. Next comes Neptune - 1 × 10²⁶. The fourth most massive planet is the gas giant Uranus, whose mass is 8.7 × 10²⁵ kg.
Next come the terrestrial planets, rocky bodies, in contrast to the gas giants with their large radius and relatively low density.
The heaviest of this group is our planet; we have already calculated its mass. Next comes Venus, the mass of this planet is 4.9 × 10²⁴ kg. After it in the ranking comes Mars, it is almost 10 times lighter - 6.4 × 10²³kg. And it closes as the planet of the smallest mass, Mercury - 3.3 × 10²³kg. Interestingly, Mercury is even lighter than two satellites in the solar system - Ganymede and Callisto.
Determining the masses of stars and galaxies
In order to find the characteristics of single star systems, the gravimetric method is used. Its essence is to measure the gravitational redshift of the star's light. It is measured by the formula ∆V=0.635 M/R, where M and R are the mass and radius of the star, respectively.
Indirectly, one can also calculate the mass of a star from its visible spectrum and luminosity. First, its luminosity class is determined using the Hertzsprung-Russell diagram, and then the mass/luminosity relationship is calculated. This method is not suitable for white dwarfs and neutron stars.
The mass of a galaxy is calculated mainly from the rotation speed of its stars (or simply from the relative speed of the stars, if it is not a spiral galaxy). The same universal law of gravity of Newton tells us that the centrifugal force of stars in the galaxy can be expressed in the formula:
Only this time we substitute the distance from the Sun to the center of our galaxy and its mass into the formula. This way you can calculate the mass of the Milky Way, which is 2.2 × 10⁴⁴g.
Do not forget that this figure is the mass of the galaxy without taking into account stars whose orbits are located outside the orbit of rotation of the Sun. Therefore, for more accurate calculations, the outermost stars of the arms of spiral galaxies are taken.
For elliptical galaxies, the method for finding mass is similar, only there the relationship between the angular size, the speed of motion of the stars and the total mass is taken.